Research
Our core premise is that what scientists really want through "data analysis" is to understand cause and effect, and ultimately, to discover how to control systems to produce a desired outcome. From an applied mathematical perspective, this desire is a wish for dynamical models that can accurately describe them. We are a group of researchers committed to teaching and learning the relevant mathematical techniques, numerical methods, and data/statistical analysis and using these methods to develop predictive models relevant to current problems in experimental and computational biology.
Research Themes
Cell Biology and Development
Gene expression is reproducibly patterned in the developing embryo on scales larger than a cell’s size and its kinetics. We use inverse-modeling techniques from applied mathematics and statistical physics to address this fundamental biological question.
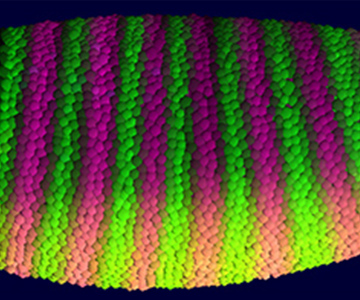
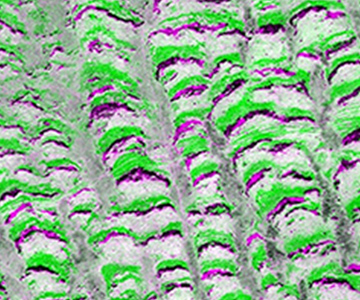
Ecology and Evolutionary Biology
Spatio-temporal patterns involving organisms in microbial communities or vegetation in arid ecosystems (shown above) can be explored via both experimental imaging and mathematical models including reaction, advection and diffusion effects.
Computational Neuroscience
Neurons form networks that can have thousands of connections per cell. Computational models can yield insight that helps explain experimental observations, and at the same time provide a testbed within which to develop new, experimentally verifiable hypotheses.
Data and Quantitative Analysis
New high throughput experimental techniques are being used to explore living systems in ways not previously possible. Researchers must know how to analyze this data and understand how to make models that are quantitatively consistent with it.